Class 11 | Physics | chapter 6 | WORK, ENERGY AND POWER | FACT/DEFINITION TYPE QUESTIONS | MCQs | NEET | JEE

 FACT/DEFINITION TYPE QUESTIONS

Question. 

No work is done if
 (a) displacement is zero
 (b) force is zero 
c) force and displacement are mutually perpendicular
 (d) All of these

Answer

(d) W = FS cos T 

 If F = 0; W = 0 

If S = 0; W = 0 &

 if T = 90°; cos 90° = 0 

 W = 0

Question. 

Work is always done on a body when
 (a) a force acts on it 
(b) it moves through a certain distance 
(c) it experiences an increase in energy through a mechanical influence 
(d) None of these 

Answer

Question.

When the force retards the motion of body, the work done is 
(a) zero 
(b) negative 
(c) positive 
(d) Positive or negative depending upon the magnitude of force and displacement

Answer

b

Hint: This is a direct question. We will make use of the formula that relates the work done, force, displacement, and the angle of displacement to solve this problem. As the retardation represents the negative sign, thus, we will substitute this value in terms of the angle of displacement to find the sign of the retardation.
Formula used:

W=FScosθ$$W=FS\cos \theta$$

Complete step-by-step solution
In the case of retardation, the work done by the force will be negative. The force that relates the work done, force, the displacement, and the angle of displacement is given as follows.

W=FScosθ$$W=FS\cos \theta$$

Where W represents the work done, F represents the force applied, S represents the displacement, and cos of angle represents the angle of displacement.
As during the retardation, the angle of displacement will be

180∘$$180{}^{\circ }$$

, so, we will substitute this value in the formula. So, we get,
 

W=FScos180∘$$W=FS\cos 180{}^{\circ }$$

Substitute value of the cos angle.

W=FS(−1)W=−FS$$\begin{array}{rl}& W=FS(-1)\\ & W=-FS\end{array}$$

The above equation represents the work done by the force. As the work is done equals the negative of the product of the force and displacement, thus, the work done by the force will be negative.
∴$\therefore$ When a force retards the motion of a body, the work is done by the force during retardation is negative.
As the work is done by the force during retardation is negative, thus, option (B) is correct.

Note: The retardation itself represents the negative sign, as it takes place in the opposite direction. The force that relates the work done, force, displacement, and the angle of displacement should be known to solve this type of problem.

Question. 

According to work-energy theorem, the work done by the net force on a particle is equal to the change in its 
(a) kinetic energy
 (b) potential energy 
(c) linear momentum 
(d) angular momentum

Answer

(a) Work done by the net force = change in kinetic energy of the particle. This is according to work energy theorem.

Question.

A light and a heavy body have equal momentum. Which one has greater K.E.? 
(a) The lighter body
 (b) The heavier body
 (c) Both have equal K.E.
 (d) Data given is incomplete

Answer

Question.

A particle of mass m has momentum p. Its kinetic energy will be 

Answer

 (d) Let the velocity of the particle be v m/s.

 Momentum of the particle (p) = mv Kinetic energy of the particle

Question.

Total ....X.... energy of a system is conserved, if the forces, doing work on it, are .....Y..... . Here, X and Y refer to
 (a) conservative, mechanical 
(b) mechanical, conservative 
(c) mechanical, non-conservative
 (d) kinetic, conservative

Answer

(b) The principle of conservation of total mechanical energy can be stated as, the total mechanical energy of a system is conserved if the forces, doing work on it, are conservative

• The law of the conservation of energy states that energy is neither created nor destroyed.
• Work can be defined as the process of energy transfer.
• There are two types of energy: potential and kinetic.
• Potential energy is energy that is stored in an object due to its position. This type of energy is not in use but is available to do work. 
• Kinetic energy is energy that is possessed by an object due to its motion. 
•  All energy is either potential or kinetic.
• Mechanical energy is a form of energy.
• The sum of an object's kinetic and potential energy equals the object's total mechanical energy. Other forms of energy include chemical, nuclear, electromagnetic, thermal and sound.

Question.

A bullet is fired and gets embedded in block kept on table. If table is frictionless, then
 (a) kinetic energy gets conserved
(b) potential energy gets conserved
 (c) momentum gets conserved 
(d) both (a) and (c)

Answer

c

(c) Only momentum is conserved. Some kinetic energy is lost when bullet penetrates the block.

Question.

From NCERT PMT - 2005

Which of the following is not a conservative force?

(a) Gravitational force

 (b) Frictional force 

(c) Spring force

 (d) None of these

Answer

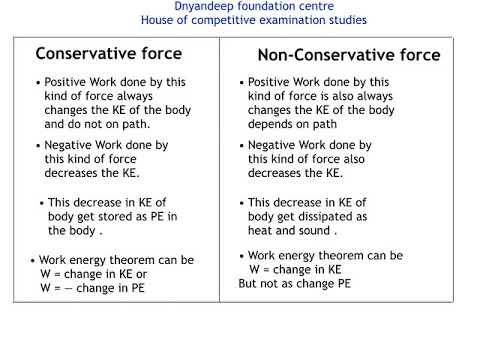
 (b) As work done by frictional force over a closed path is not zero, therefore, it is non-conservative force. 

Question.

If a light body and heavy body have same kinetic energy, then which one has greater linear momentum?
 (a) Lighter body 
(b) Heavier body 
(c) Both have same momentum
 (d) Can’t be predicted

Answer

Question.

A light and a heavy body have equal momentum. Which one has greater K.E.? 
(a) The lighter body 
(b) The heavier body
 (c) Both have equal K.E. 
(d) Data given is incomplete

Answer

Question.

The time rate of change of kinetic energy is equal to

Answer

Question.

The rate of change of kinetic energy is $n$ times ($n$ is constant with appropriate dimension) the velocity at any moment of a particle of mass $m$, which starts moving in a straight line. The constant acceleration with which particle is moving is 

A

$mn$

B

$\frac{m}{n}$

C

$\frac{n}{m}$

D

mn1​

Answer

Correct option is

C

$\frac{n}{m}$

$K=\frac{1}{2}m{v}^2$

$$\begin{gathered} \frac{dK}{dt}=mv\times \frac{dv}{dt}=m\frac{dv}{dt}v=mav, \\ \Rightarrow mav=nv \end{gathered}$$

$ma=n,a=\frac{}{}$

Question.

Using the equation for kinetic energy: $k=\frac{1}{2}m{v}^2$ Find the instantaneous rate of change of the kinetic energy of a $1500kg$ car which has a velocity of $80\frac{m}{s}$ and an acceleration of $10\frac{m}{s^2}$?

a)1.2×10^6 JS^1

b)1.2×10^3 JS^1

c)1.2×10^4 JS^1

d)1.2×10^5 JS^1

Answer

correct option (a)

Kinetic energy $KE=\frac{1}{2}m{v}^2$
Instantaneous rate of change of KE is its differential with time
$\stackrel{.}{KE}=\frac{d}{dt}\left(\frac{1}{2}m{v}^2\right)$
$\Rightarrow \stackrel{.}{KE}=\frac{1}{2}m\cdot 2v\cdot \frac{dv}{dt}$
$\Rightarrow \left(\stackrel{.}{K}E\right)=m\cdot v\cdot a$
Where $\stackrel{.}{v}=a$, acceleration.
Inserting given instantaneous values we get

$\Rightarrow \stackrel{.}{KE}=1500\cdot 80\cdot 10$
$\Rightarrow \stackrel{.}{KE}=1.2\cdot {10}^{6}J{s}^{-1}$

Question.

Two bodies of different masses are moving with same kinetic energy. Then the ratio of their momenta is equal to the ratio of their
 (a) masses 
(b) square of masses
 (c) square root of masses
 (d) cube root of masses

Answer

Question.

NEET(UG) 2017- A spring of force constant k is cut into lengths of ratio 1:2:3. They are connected in series and the new force constant is k'. Then they are connected in parallel and force constant is k”. Then k': k" is :-
 (1) 1 : 9                           (3) 1 : 14 
(2) 1 : 11                          (4) 1 : 16

 

Answer

Important concept-Work Done by ideal springs

Example: A spring obeying the linear law $F=-Kx$ is first compressed by
$10$ cm and the work done is $W_1$. Next it is compressed by
another $10$ cm, the work done now is $W_2$, then what is the value of $W_1:W_2$ ?
Solution:
$W_1=\Delta (P.E)$
$=\frac{1}{2}K(x{{}_2}^2-x{{}_1}^2)$
$=\frac{1}{2}K(10^2-0^2)$
Similarly,  $W_2=\frac{1}{2}K(20^2-10^2)$
So, $W_1:W_2=100:300$
$=1:3$

Question.

A spring with force constant k is initially stretched by x1 . If it is further stretched by x2 , then the increase in its potential energy is

Answer

Video Youtube

Question.

The speed of an object of mass m dropped from an inclined plane (frictionless), at the bottom of the plane, depends on:
 (a) height of the plane above the ground 
(b) angle of inclination of the plane 
(c) mass of the object 
(d) All of these

Answer

(a) If an object of mass m is released from rest from top of a smooth inclined plane, its speed at the bottom is (Root 2gh) , independent of angle T and mass.

v2-u2=2gh

Question.

An object of mass m is released from rest from the top of a smooth inclined plane of height h. Its speed at the bottom of the plane is proportional to

A

m0

B

m

C

m2

D

m−1

Answer

Answer

A

Solution

Let v be the speed of the object at the bottom of the plane.
According to work-energy theorem
W=ΔK=Kf−Ki${\displaystyle W=\Delta K=K_f-K_i}$
mgh=12mv2−12mu2 (∴u=0)${\displaystyle mgh=\frac{1}{2}m{v}^2-\frac{1}{2}m{u}^2(\therefore u=0)}$
mgh=12mv2orv=2gh−−−√${\displaystyle mgh=\frac{1}{2}m{v}^2\text{or}v=\sqrt{2gh}}$
From this expression, it is clear that v is independent of the mass of an object.

Question.

If two particles say proton are brought near one another, the potential energy of the system will
 (a) increase
 (b) decrease 
(c) remains the same 
(d) equal to the K.E

Answer

(a) This is because in bringing two protons closer, work has to be done agains the force of repulsion. This is stored up in the form of potential energy. However, the potential energy will decrease when a proton and an electron are brought nearer.

Question.

Work done by a conservative force is positive if 
(a) P.E. of the body increases 
(b) P.E. of the body decreases 
(c) K.E. of the body increases 
(d) K.E. of the body decreases

Answer

(b)

Hint: When a body is displaced in the direction of the force then it is known as positive work on a body. This causes the movement of objects in a direction either toward the center of force or away from the center of force. Due to this the separation between the body and center of force increases or decreases. This causes variation in the potential energy and kinetic energy of the body. Thus, keep the total energy constant. Use these basics to answer this question.

Complete step by step answer:
When the conservative force pushes or displaces the body in the direction of the force, the conservative force does a positive work on the body. Thus, the body moves towards the center of force. This causes a decrease in the separation between the body and the center of force. Thereby, decreasing the potential energy of the body and increasing the kinetic energy of the body.
Hence, when a conservative force does positive work on a body, the potential energy decreases.

So, the correct answer is option B i.e. the potential energy decreases.

Note:
When the conservative force pushes the body in the opposite direction of the force, the conservative force does a negative work on the body. This causes the body to move away from the center of force. Thus, the separation between the body and the center of force increases. Hence, when a conservative force does a negative work on the body, the potential of the body increases. As the potential energy increases, kinetic energy decreases.

Question.

The potential energy of a system increases if work is done 
(a) upon the system by a non conservative force 
(b) by the system against a conservative force 
(c) by the system against a non conservative force 
(d) upon the system by a conservative force

Answer

(d) When work is done upon a system by a conservative force then its potential energy increases.

Question.

For a conservative force in one dimension, potential energy function V(x) is related to the force F(x) as

Answer

(a) Conservative force is negative gradient of potential F(x) = –dV(x)/ dx

Gradients and Partial Derivatives(5min Video)

Question.

If stretch in a spring of force constant k is doubled then the ratio of elastic potential energy in the two cases will be
 (a) 4 : 1 
(b) 1 : 4 
(c) 2 : 1 
(d) 1 : 2

Answer

Question.

Answer

uestion.

Question.

Answer

Question.

Answer

Question.

Answer

Question.

Answer

Question.

Answer

Question.

on